Mathematics through common core is seen in mixed ways. While some people believe it will advance learning for students and help them as concepts get harder, others think common core is just to hard for students. In the post below, I will analyze two sources about common core mathematics from the scholarly journal, LexisNexis.
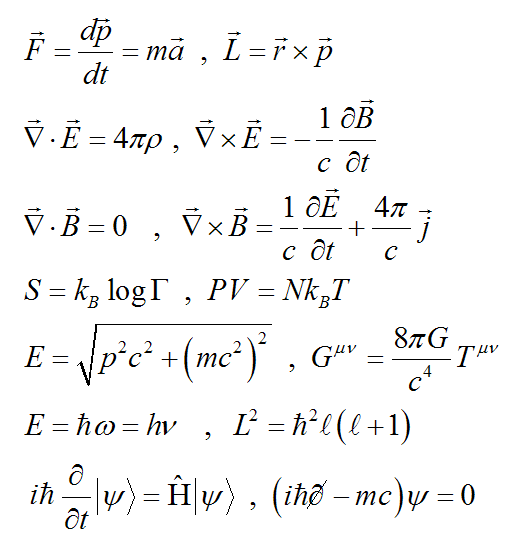 |
| MathKnight. "Physical Equations." 2/1/2008 via Wikipedia. Public Domain License |
The first source is written by Solomen Friedberg, and he argues that common core is clear, concise, and helpful in his paper, titled, "It's not fuzzy math. It's fluency; A mathematician's view about Common Core's new standards." Friedberg published this to LexisNexis on September 16, 2014. In his paper, Friedberg discusses how common core works, and goes on to show how mathematical societies endorse it as well (like the American Mathematical Society). He clearly states that he supports common core, and that he believes it is a clear step in the future of education because it encourages learning the concepts, not memorization of rules to solve problems. Friedberg is trying to convince people that common core is the best option for education, and he outlines why by using examples and backing himself up with other, credible, sources of information.
In Jordan Ellenberg's paper, "Meet the New Common Core," she describes the new standards and tests some states are replacing common core with. She describes it, and then points out that it is almost the exact same thing as common core. She goes on to describe how common core works, similar to Solomen Friedberg, and concludes that, while there may be problems with common core, those problems have existed before, during, and will continue to exist after common core. In her last paragraphs, Ellenberg continues to argue that common core is necessary, and that it only changes the emphasis of mathematical learning, no matter the name states give it. It seems as though she tries, more so than Friedberg, to inform readers of the changes and how they aren't actually all that different than common core. Her conclusion is her persuasive part, where she attempts to convince readers that common core is not as bad as it seems, and that most people just don't understand it.
In Jordan Ellenberg's paper, "Meet the New Common Core," she describes the new standards and tests some states are replacing common core with. She describes it, and then points out that it is almost the exact same thing as common core. She goes on to describe how common core works, similar to Solomen Friedberg, and concludes that, while there may be problems with common core, those problems have existed before, during, and will continue to exist after common core. In her last paragraphs, Ellenberg continues to argue that common core is necessary, and that it only changes the emphasis of mathematical learning, no matter the name states give it. It seems as though she tries, more so than Friedberg, to inform readers of the changes and how they aren't actually all that different than common core. Her conclusion is her persuasive part, where she attempts to convince readers that common core is not as bad as it seems, and that most people just don't understand it.
No comments:
Post a Comment